Misconceptions in Math

Upper elementary teachers frequently see their students’ misconceptions in math. Have you ever taught a math concept where you had to spend as much time undoing misconceptions as you do teaching new concepts? We often inadvertently teach misconceptions when we offer our students tricks and shortcuts to math concepts. This issue isn’t isolated to any one group of teachers or grade levels. In fact, research shows a significant drop in math test scores as students reach middle school and beyond. Of course, there many many factors in the decline of test scores, but I can’t help but think of the possibility that students are approaching higher levels of math with an abundance of math misconceptions. I recently read Math Misconceptions, which you can find here, and it solidified my thinking that there are things we can do as elementary educators to prevent misconceptions in math.

This blog post shares eight phrases teachers frequently say that may be responsible for causing misconceptions in math. I know that I’m guilty of using more than one, and I’m sure that I’ve missed some phrases too.

1. The Equal Sign Means “The answer is…”

Many students view the equal sign as a symbol that means “the answer is” or “ta-da”. Instead, the equal sign in math means what is to the right and left of the symbol have the same value. Understanding the equal sign as a relational symbol becomes more important when students begin solving equations with a missing addend, minuend, subtrahend, factor, dividend, or divisor when the equal sign is not in a standard position  To promote an understanding of the equal sign, students need exposure to a variety of equation types (3 = 8 − 5; 2 + 3 = 1 + 4; 9 − 3 = 6). Students need an understanding of the equal sign to work on higher-level math problems such as word problems and algebraic equations. If students do not learn the correct interpretation of the equal sign performing other higher-level math calculations will become more difficult as school progresses.

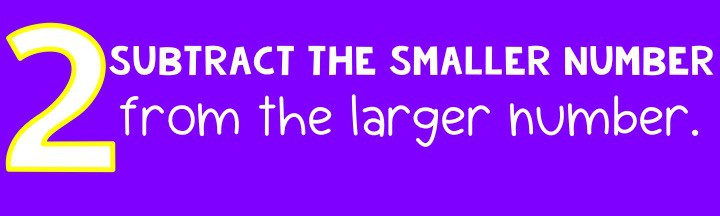
2. Subtract the Smaller Number from the Larger Number

A common misconception in math is when learning subtraction, students often learn to write the big number first. However, this is not always true. While this subtraction rule may work for students in elementary school, this is not the case when students begin to add and subtract positive and negative integers. This rule may also create a problem when students subtract multi-digit numbers. Students often overgeneralize previous learning and subtract the smaller number from the larger one digit-by-digit. For example in the problem 453-172. Students may correctly subtract 3-2, but when they reach the tens place, they subtract 7-5. Students need to be taught that subtraction is not commutative and the importance of order in subtraction.

3. Multiplication Makes Numbers Bigger

Most of the time in the elementary classroom, multiplication makes numbers bigger. However, this is a common misconception in math. Once students reach fifth grade and are not exclusively multiplying whole numbers, this is not always true. For example in the problem 0.5 x 0.2 = 0.1, the product has a smaller value. Students must develop multiple strategies to support understanding of multiplication and to develop number sense that extends beyond whole numbers. A great way to begin a multiplication of fractions lesson would be to ask students if it’s true that multiplication always makes numbers bigger.

4. Misconceptions in Math – Division Makes Numbers Smaller

Just as multiplication does not always make numbers larger, the same can be said for division. Division does not always make numbers smaller. For example in the problem 6 ÷ ½ = 12, the quotient is larger than the dividend. It’s amazing that students will be solving that type of problem as early as fifth grade, and as a third and fourth grade teacher, I want my students prepared for that type of math reasoning. Even though I don’t necessarily teach that concept, I don’t want to create rules or frame concepts that will lend themselves to confusion in the future.

5. Always Divide the Larger Number by the Smaller Number

Students are often told that they always divide the larger number by the smaller number. However in fifth grade, this is not always the case. For example in the problem 4 ÷ 6 = 2/3, the dividend is smaller than the divisor. When this concept “sticks”, it is extremely difficult to undo later. An example that students can understand is having 2 pounds of cat food that has to be split among the 4 cats. Working on equal sharing situations like this helps students develop the number sense to ultimately handle operations with fractions. One way to avoid the misconception of putting the larger number first is to focus instead on the parts of an equal sharing problem. Is anyone else feeling the need to offer some words of encouragement to the fifth grade teachers at your school?

6. When You Multiply by 10, Add Zero to the Number

A major part of third and fourth grade math is teaching students how to multiply by multiples of ten. Teachers often tell students that when you multiply a number by ten, just add a zero to the end of the number. However, once again, this isn’t always true and leads to misconceptions in math. When students multiply decimals by ten and powers of ten, they do not add a zero to the end of the number. You can see an example in the problem 7.47 x 10 ≠ 7.470. Not only does the rule lead students to misconceptions, but it also prevents students from developing a deeper understanding of our base-ten system. Students should learn that when multiplying by 10 or 100, each digit is shifted to the left on a place value table, because they are adding another place to the number. You can learn more about how I teach multiplying and dividing by multiples of ten in this blog post.

7. In a Fraction, the Big Number Always Goes on the Bottom

Many times, we tell student to always write the big number on bottom in fractions. Not only does this rule prevent authentic understanding of the concept of fractions, it is not an accurate rule. When students write improper fractions in fourth grade, the numerator is greater than the denominator. Rather than teaching the rule, students should develop an understanding of a whole, numerator, and denominator. Students tend to manipulate fractions by rote rules and memorization, rather than try to make sense of the concepts and procedures. During one of my fraction lessons last year, students made the generalization that if the numerator is larger than the denominator, the fraction is greater than one whole. This generalization was generated by students through hands-on exploration of fractions-not by me stating the rule.

8. Misconceptions in Math – If it’s a Rectangle, it Can’t Be a Square

Students are often taught that the categories of shapes cannot overlap. For example, a square cannot also be a rectangle. Once students enter third grade and begin to study the hierarchy of quadrilaterals, these statements do not apply. A square is a special type of rectangle, and a square is also a special type of rhombus. Then to complicate things even further, a square, rectangle, and rhombus are all types of quadrilaterals. Students have to be flexible in their reasoning about math terms and concepts.

Misconceptions in Math Keywords

I couldn’t write this blog post without mentioning keywords. There are some schools of thought that are totally and completely against key words. I understand the reasoning, but I don’t think that teaching math keywords has to be a completely bad thing. I do believe it’s possible for students to overly rely on keywords or to over generalize key words. However, I also recognize that keywords can become problematic when students begin to solve multi-step word problems, because they must decide which keywords work with which component of the problem.

Even so, I believe keywords can play a positive role in the reading comprehension aspect of word problems. Students must understand the vocabulary and meaning of keywords in the problem. So while I continue to introduce them to my students, I do not introduce them in isolation. When students are taught the underlying structure of a word problem, they not only have greater success in problem solving but can also gain insight into the deeper mathematical ideas in word problems. This is why I always make types of word problems a major part of my math instruction. I’ve added types of word problems for Addition and Subtraction and Multiplication and Division to my Math Reference Notes.

I’ve also created a small Types of Word Problems bulletin board in my classroom for an easy reference. As I introduce and teach the different types of word problems, I have students complete a different page in their Types of Word Problems booklet. Throughout the year, I also add various types of word problems to students’ work station activities.