Systematic Instruction

Systematic instruction intentionally builds students’ knowledge over time toward a specific learning outcome. This post continues the blog series on how to support students who struggle and shares strategies to incorporate systematic instruction for students who have difficulty in math.

Systematic Instruction – Review

I imagine that the review component of systematic instruction isn’t shocking to any teacher! It’s essential to review previously learned content to ensure students maintain understanding of concepts and procedures. During the review, it’s important to give students a variety of problems to avoid having students overgeneralize new concepts or procedures. For example, after introducing subtraction with regrouping, include problems that do not require regrouping. This will help prevent students from regrouping all problems. Or, when teaching multi-digit division include problems with 2, 3, or 4-digit quotients.

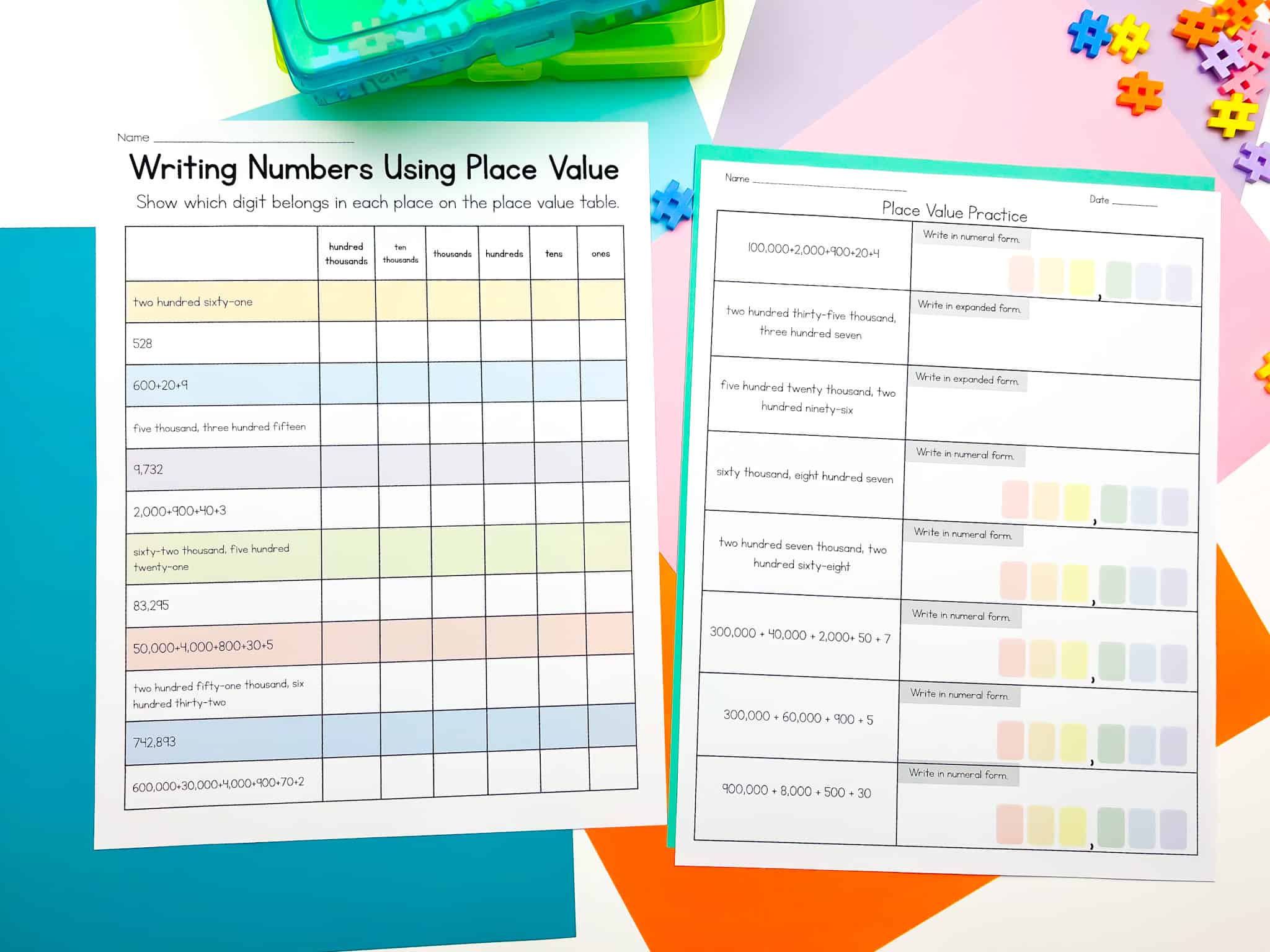
In my remediation units (Place Value), all lessons include a review component. The review includes content from previously taught skills and concepts, as well as continuously changes to adjust for new content.

The photo above shows how the review is included in the lesson plan. You can see how it’s broken down into parts. Below is an example of a typical math review.

Accessible Numbers

When introducing new content, use accessible numbers to support learning. During our initial instruction, the numbers we use should be easy for students to work with. This allows students to focus on the new concept, rather than on difficult calculations. For example, when teaching division, students may benefit from primarily working with divisors of 5 and 3. When students have a grasp of the concept, systematically add other numbers as the divisor.

You can see in the example from my place value resource that I initially don’t include numbers with zero. I first make sure students understand the general concept, and then I begin systematically including zeros in the numbers.

Systematic Instruction – Incremental Learning

We should sequence instruction so that the math students’ learning builds incrementally. Students who struggle in math often need to focus on smaller tasks that are needed to solve complex problems. When students become comfortable with smaller tasks, they can eventually connect those tasks to more complex problems. This is quite different from student-led inquiry based instruction as it is much more teacher-led and direct. However, some students need this style of lesson.

In the photos below, I used some of my rounding lessons as an example of incremental learning. Students who need specialized instruction often need the process broken down into small steps over the course of multiple lessons.

Step 1

I first teach students how to find numbers on a number line. It’s so easy and tempting to overlook this, but you may be surprised at how little students understand number lines. Ideally, they will have worked with them consistently, but they may be brand new (or rarely used) to many students.

Step 2

Then students learned how to find benchmark numbers on number lines. When I started isolating benchmark numbers, I was shocked at how much trouble this gave my students. It helped me to understand why rounding was always so tricky. If they don’t understand benchmark numbers, rounding is just a memorized process.

Step 3

After those parts of rounding have been taught, it’s time teach students how to round with a number line. To keep instruction systematic, I begin with the tens place and gradually incorporate different place values.

Of course, this isn’t a post on teaching students how to round. (Although, I do have those posts:) Instead, I hope it shows an example of incremental instruction.

Visual and Verbal Supports

As we work with students, we must continuously provide visual and verbal support. Our verbal supports include prompting and questioning that helps students remember prior learning or focus on the math they are currently doing.

Students also benefit from visual supports such as pictures, diagrams, or hints as reminders about specific concepts. I often include visual supports directly on students’ tasks. This support can gradually be reduced until it is no longer needed.

Systematic Instruction – Feedback

Immediate feedback is critical for systematic instruction because it prevents students from developing misunderstandings. As students work, ask they to explain their understanding and provide feedback and guidance to their response. If they solve problems incorrectly, ask questions to identify misconceptions and determine the cause of the error. As we ask questions, try to structure questions to help students self-identify where their thinking went wrong.

This is a personal area of growth for me. I’ve found myself slipping into a role of “validator of right and wrong”. My goal is to teach students to validate themselves.

Learn More

You can learn more about teaching students who struggle in math with the posts linked below.

Systematic Instruction

Systematic Instruction – Review

Accessible Numbers